
Pressure Vessel, Nonimpact Design
Based on the classical Venturi design, nonimpact Venturi meters are those that sense only axial components of the line fluid’s velocity profile; nonaxial vectors are considered insignificant in normalized piping. The resulting flow meter has a discharge coefficient (CD) that approaches a value of one, is not a function of fluid velocity and beta ratio (d/D), and is constant above a given threshold pipe Reynolds number. In general, designs that utilize this flow metering mechanism are less sensitive to upstream pipe fittings than those designs that employ other types of pressure sensation.
The LVM nonimpact design conforms to all those characteristics that make Venturi meters the preferred flow meter when accuracy, reliability, and low permanent pressure loss are required.

Insert-Type, Impact Design
This type of design senses a nonaxial velocity component in the high pressure sensation. As a result, the meter discharge coefficient is dependent on the beta ratio; however, Cd is not a function of line velocity and is still constant above a given threshold pipe Reynolds number.
The LVM impact flow meter is a cost-effective alternative to the nonimpact pressure vessel design. While slightly more sensitive to upstream piping than the nonimpact design, these insert-type Venturi meters provide the same accuracy, reliability, and low-pressure loss as that of the nonimpact design.
The value of the CD indicates a very efficient metering shape; further, the CD shows no velocity dependence. Only pipe Reynolds number (RD) is a concern: If the pipe Reynolds number is greater than 75 000, the substantiated uncertainty of the LVM design is better than ± 0.50%; for RD < 75 000, CD is known and repeatable. Contact Wyatt Engineering for further information.
Installation Effects
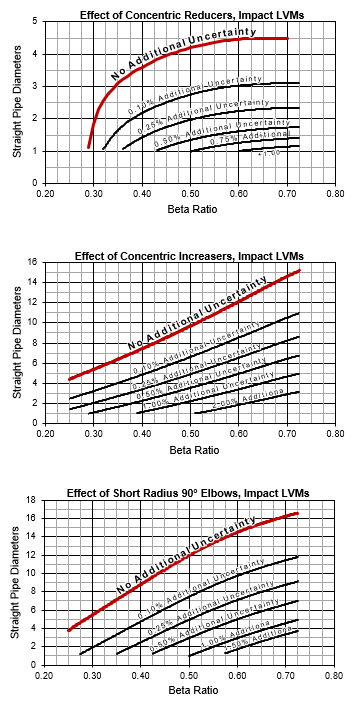
The curves shown above summarize the results of flow tests on impact LVM flow meters. In this case, the label, “impact,” indicates the high pressure tap senses nonaxial velocity components. This is due to the location of the high-pressure tap located either behind the meter inlet cone or at the intersection of the converging section and the upstream pipe. Meters employing this type of high-pressure sensation are sometimes, erroneously, referred to as using “full or partial Pitot effects.” Due to the nonaxial velocity component that influences the high-pressure sensation, the meter discharge coefficient (CD) is dependent on beta ratio, independent of line velocity, and still constant for pipe Reynolds numbers greater than about 75 000. The impact design typically refers to insert-type meters, the most common of which are Wyatt Engineering models LVM-IF, LVM-IL and LVM-IP.
Energy Considerations
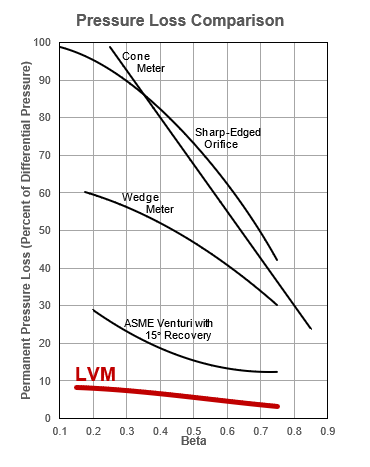
Energy loss, called specific headloss, is typically expressed in terms of pounds per square inch, inches of water column, kilopascals, etc. Losses for some different meter types are shown in Figure 1. In terms of generalized units, however, the true description is, “Units of Energy per Units of Mass Flow.”
Consequently, the specific headloss of a meter, fitting, etc., represents an ongoing energy expense, “the cost of doing business,” and it is everyone’s duty to minimize that cost. For reference, an LVM sized for the same differential pressure consumes is less than one-eighth the energy of an orifice plate.
Pressure measured at a given point that does not have a nonaxial velocity vector is an indirect indication of the potential energy content of the flowing fluid at that cross section. Consequently, pressure drop is a measure of the energy dissipated between two points.
Installation Effects (cont.)
The differential pressure produced by the LVM design is an indication of the difference in the kinetic energy of the line fluid between the high and low-pressure tap cross sections. Due to differing velocity profiles, a given flow rate can possess different kinetic energies, and thereby introduce errors in the indicated flow rate. This is the essence of the study of installation effects and allows for the calculation of installed uncertainty.
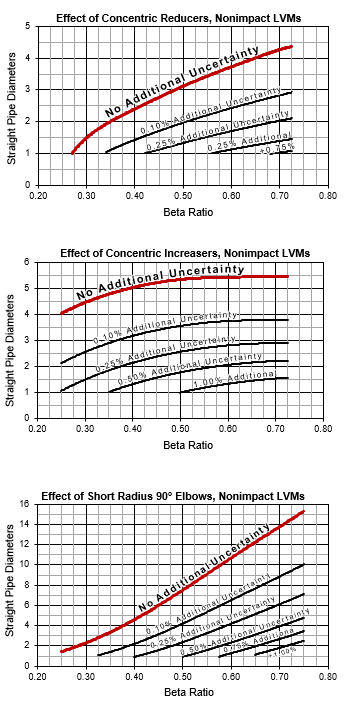
These curves summarize the results of flow tests on nonimpact LVM flow meters. The “nonimpact” term reflects the condition that neither the high nor the low-pressure tap senses nonaxial velocity components. This refers to meters commonly (but technically incorrect) labeled “static tap” design. The most common Wyatt Engineering model numbers appropriate for these data are: LVM-B, LVM-CI, LVM-EV, LVM-F, and LVM-U.
The test results show a clear relationship between the installation error function, EI , and β, the diameter ratio:
EI=0 .
In other words, meter sensitivity to an upstream disturbance decreases with decreasing diameter ratio (d/D), or the smaller the beta, the smaller the effect of upstream piping. Note that a similar relationship exists for orifice meters, flow nozzles, and nozzle Venturi meters. Simple enough, but here’s the rub:
∆H=∞ ,
the smaller the beta ratio, the higher the permanent pressure loss, ΔH. While the desire is to minimize both the specific headloss and installed uncertainty, sometimes increased permanent pressure loss is the price paid for improving the installed accuracy.
Flow patterns that change the behavior of the discharge coefficient can be caused by pipe fittings, Reynolds number, and/or pipe surface/diameter irregularities. From flow testing, we have learned that non-rotational, irregular flow patterns, that is, those caused by reducers, single elbows, etc., are self-attenuating, while irregular flow patterns that are rotational, for example, those caused by two elbows direct-coupled in perpendicular planes, are relatively stable and users should consider flow straighteners in such cases.
To determine the installed uncertainty, add the installation effect to the LVM uncertainty. The standard uncertainty is ±0.50% for Wyatt’s QS9001 calibrated meters, and (generally) ±0.25% for independently flow calibrated meters. These uncertainties are stated at a 95% confidence interval and should not be applied to other meter designs or models.
With respect to downstream piping requirements, pipe fittings, valves, etc. may be direct-coupled to the outlet of a Liberty Venturi Meter without affecting the accuracy. Note that some meters’ recovery sections are truncated, so direct-coupling a butterfly valve may, in some instances, cause the valve disk to interfere with the meter outlet and full valve disk movement may not be possible. Let us know if this configuration is required and the meter outlet will be modified accordingly. It should be noted also that the pressure loss of the direct- or close-coupled valve or pipe fitting will be somewhat higher than is calculated assuming a blunt velocity profile. If this is a concern, please contact Wyatt Engineering for further assistance.
Uncertainty Substantiation
The method outlined on this page is the correct approach; anything else is sales talk.
Uncertainty without Flow Calibration
The vast majority of Liberty Venturi Meters are provided without flow calibration. This is due to our ability to substantiate the uncertainty of the LVM to within ± 0.50% of indicated flow rate for pipe Reynolds numbers greater than
75 000.
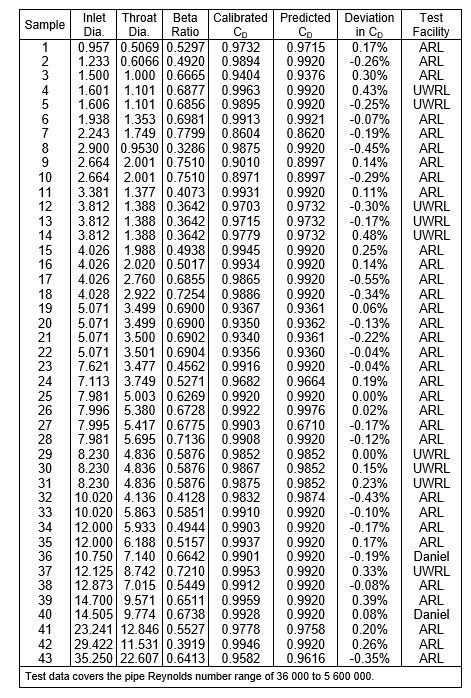
For the LVM design, there is a “true” discharge coefficient (CD). The true CD can be estimated only through repeated observations, but those observations (flow calibrations) will have uncertainties associated with them. The observed CD, therefore, equals the true CD plus an inevitable uncertainty band. The error on the estimated discharge coefficient, CD, approaches zero as the number of flow calibrations approaches infinity.
Since there are not an infinite number of flow calibrations, we must consider the contribution of the finite sample size to the combined uncertainty. Note that this allowance, historically referred to as precision, is often neglected in many uncertainty calculations provided by the ill-informed or inexperienced. This consideration is necessary and cannot be ignored because it accounts for the finite size of the sample.
For the calibrations shown, the standard uncertainty of the data is ± 0.243% and consideration for the small sample is
± 0.037%. Together, these provide a combined uncertainty of ± 0.246%, which, in turn, results in an expanded uncertainty of ± 0.482% at a 95% confidence interval.

Uncertainty is a calculated value, not an opinion.